

To determine the ideal tilt for your application, the following information is needed:
-
whether you're optimizing your array for maximum summer, winter, or annual power generation
-
the size of your modules and whether they will be configured in portrait or landscape orientation
-
the latitude of your location
First off, let's go over the different options for tilt optimization. Generally in an off-grid system, you want to optimize your array for winter. This is when the least amount of sunlight is available and you need to capture as much of that irradiance as possible. Because we know that a module captures the highest amount of sunlight when it is directly perpendicular to the sun and that the sun hangs lower in the sky in winter, modules in this instance need to have a higher than normal tilt.
Citation for Photovoltaics Design and Installation Manual from Solar Energy International
In a grid-tied system, tilts are generally configured according to whether or not the application is operating under Time Of Use (TOU) utility rate structures. An application under TOU is likely best served by optimizing for summer (latitude minus fifteen degrees). This is due to the increased payback from the utility during peak hours (ie summer afternoons) when your array's production is at its highest. Alternatively, a grid-tied application not operating under TOU is generally best served by optimizing for maximum annual production (latitude).
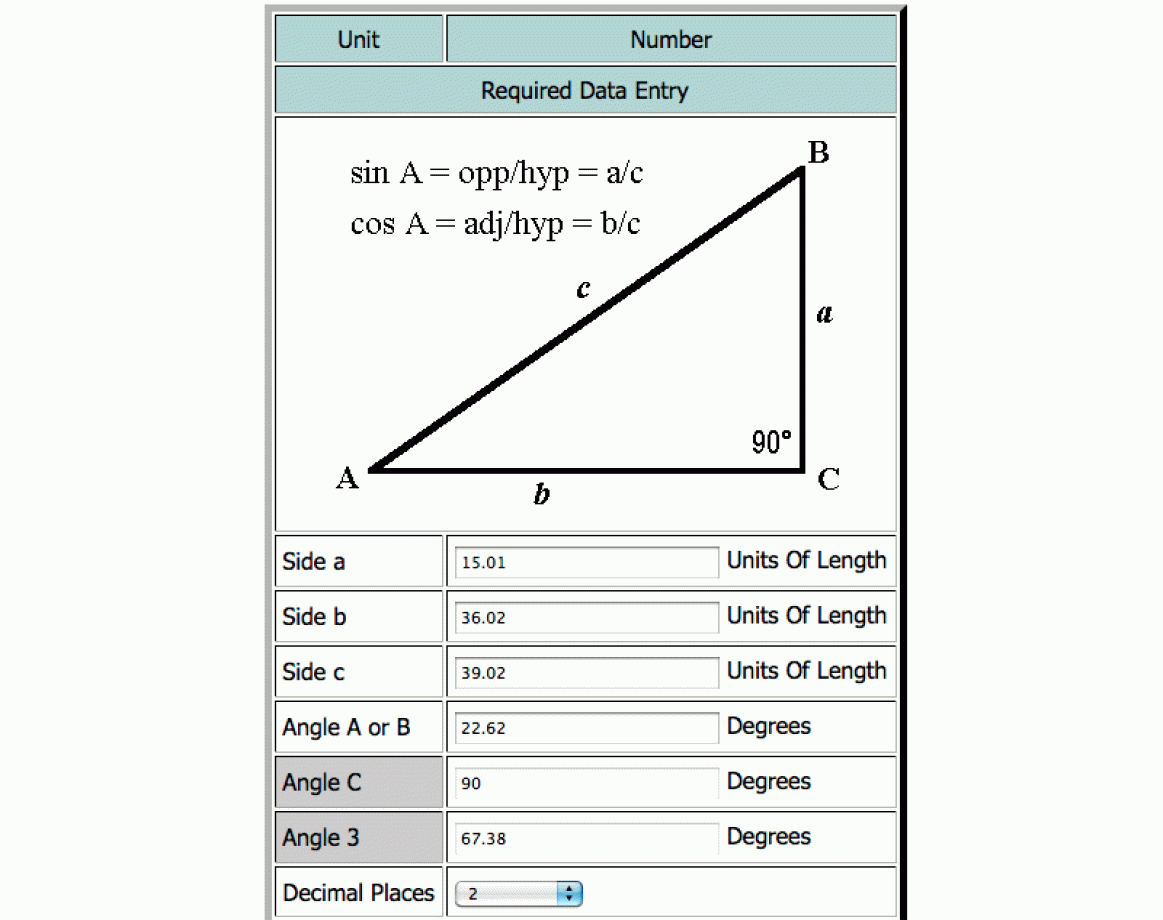
Because we are using landscape orientation, we'll use the module width of 39.02 inches for our calculations. (Alternatively, in portrait orientation we would use the module length of 65.5 inches.) Next, we need to calculate the length of the tilt leg needed to achieve an angle of 22.62 degrees with this module. A great resource to do this can be found at the following link: Right Triangle Angle and Side Calculator.
We know the length of side c is 39.02 inches and that Angle A or B is going to be 22.62 degrees. From this we can calculate the value of side a, which in this case is 15.01 inches. Thus, we know that for this application we need a tilt leg that is 15” long.